A pendulum is one of the most common items found in most households. It is a device that is commonly found in wall clocks. This article will throw light on this particular device and its functioning. After that, students will be able to easily understand how it operates and the reason behind its harmonic motion. Learn pendulum formula here.
Definition
A pendulum is essentially a weight that is hung from a fixed point. It is placed in such a way that it allows the device to swing freely to and fro. The pendulum bob of a simple pendulum is treated as a point mass. Further, the string from which it’s hanging is of negligible mass.
If you look at it from the perspective of physics, you will find these simple pendulums quite intriguing. This is so because they serve as a great example of simple harmonic motion, which is much similar to rubber bands or springs.
Pendulum Equation
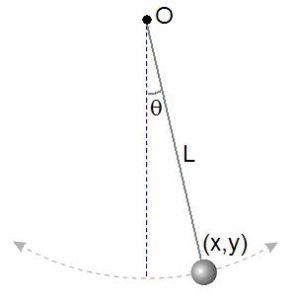
There are a lot of equations that we can use for describing a pendulum. Firstly, we have the period equation which helps us calculate how long the pendulum takes to swing back and forth. We measure it in seconds. Thus the period equation is:
T = 2π√(L/g)
Over here:
T= Period in seconds
π= The Greek letter Pi which is almost 3.14
√= The square root of which we include in the parentheses
L= The length of the rod or wire in meters or feet
G= The acceleration due to gravity (9.8 m/s² on Earth)
Next up, we have the frequency equation. This calculates the numbers of times a pendulum swings back and forth within a second. We measure that in hertz. Thus, the frequency equation is:
f = 1/T
f = 1/[2π√(L/g)]
Over here:
Frequency f is the reciprocal of the period T:
Further, we have the length of the wire. You can easily find the length of the wire or rod for a specified frequency or period. Have a look at the equation given below to know more:
f = [√(g/L)]/2π
2πf = √(g/L)
So, when you have this, you will need to square both sides of these equations. That results in:
4π2f2 = g/L
When you solve for L, you will get:
L = g/(4π2f2)
Similarly, the length of the wire for a given period is:
T = 2π√(L/g)
Then, you after squaring both the sides we get:
T2 = 4π2(L/g)
Thus, when you will solve for L, you will get:
L = gT2/4π2
Solved Example on Pendulum Formula
Question– A pendulum’s length is 4 meters. It completes one full cycle of 0.25 times every second. The maximum displacement that the pendulum bob reaches is 0.1 meters from the center. Find out the time period of the oscillation? And what is the displacement after 0.6 seconds?
Answer– To begin with, make sure to write down the information which you already know. So, by far, we already know the length of the pendulum (L= 4 meters). Then, the pendulum’s frequency is 0.25 (f- 0.25). Similarly, the amplitude or maximum displacement is 0.1 and time is 0.6 (A= 0.1 and t=0.6). Finally, the acceleration due to gravity, as always is 9.8 (g=9.8). So, you need to find T.
In order to find T, you need to simply plug numbers into this equation and solve it accordingly. So, you have the equation of 2π
2π
2π × 0.64
2 × 3.14 × 0.64 = 4.01
Therefore, the time period of the oscillation is 4.01 seconds.